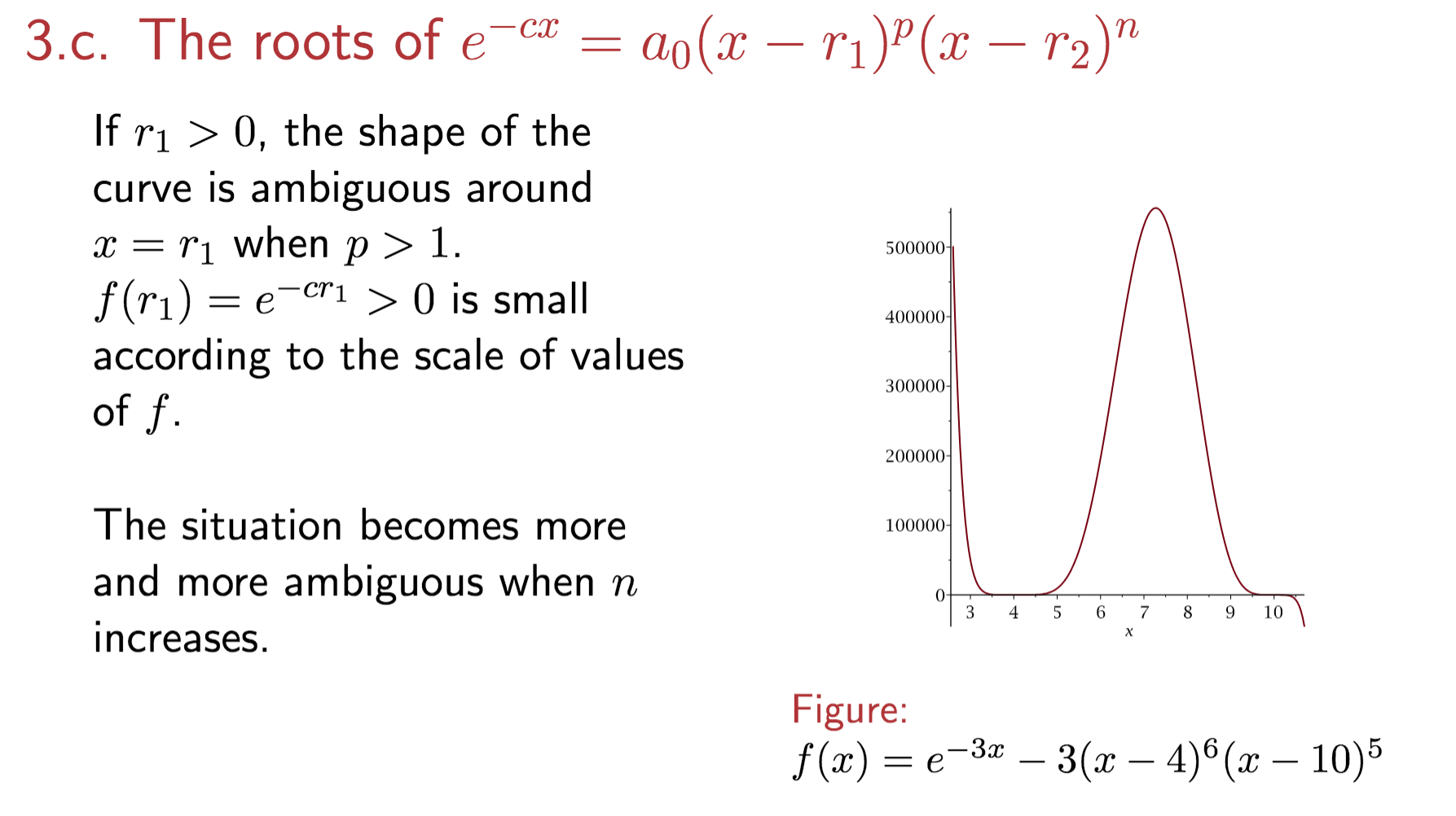
What is it about?
Herein, we use Hardy’s notion of the “false derivative” to obtain exact multiple roots in closed form of the transcendental-algebraic equations representing the generalized LambertW function. In this fashion, we flesh out the generalized Lambert W function by complementing previous developments to produce a more complete and integrated body of work. Finally, we demonstrate the usefulness of this special function with some applications.
Featured Image

Photo by G-R Mottez on Unsplash
Why is it important?
This is the bees knees of this wonderful generalization of the Lambert W function. It is a universal mathematical function that expresses many solutions and properties of quantum mechanics and relativity.
Perspectives

This function is ubiquitous i.e. universal in applications in Science and Mathematics. Since its publication, more applications have been found. The generalized Lambert W function warrants implementation in e.g. a computer algebra system.
Dr Tony Cyril Scott
RWTH-Aachen University
Read the Original
This page is a summary of: Fleshing out the generalized Lambert
W
function, ACM Communications in Computer Algebra, August 2016, ACM (Association for Computing Machinery),
DOI: 10.1145/2992274.2992275.
You can read the full text:
Resources
Contributors
The following have contributed to this page