What is it about?
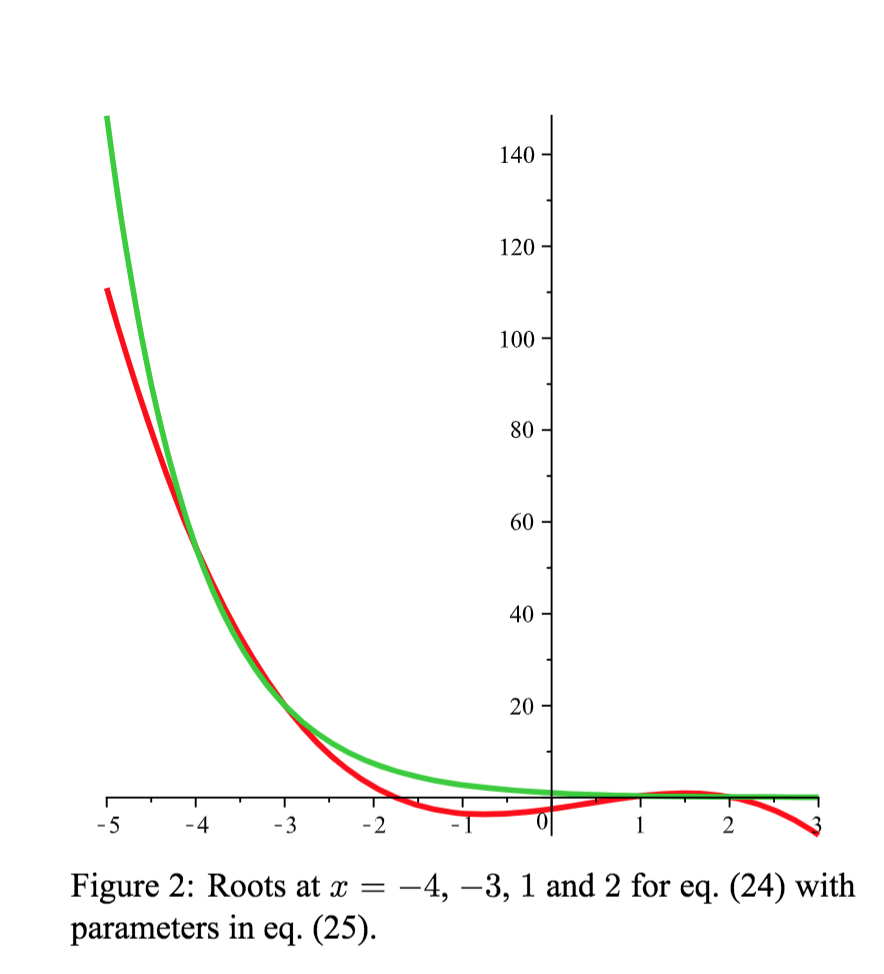
Part II on the Generalized Lambert W function: This paper presents means and methods for obtaining the real roots for the Generalized Lambert Function for real parameters when the polynomial part are quadratic or cubic polynomials in a number of viable scenarios.
Featured Image

Photo by Dimitry B on Unsplash
Why is it important?
This paper is important in working out the floating point attributes and completeness of solutions for a regime of Generalized Lambert W functions
Perspectives

This contributes in making the Generalized Lambert W function feasible for e.g. an implementation into a Computer Algebra System.
Dr Tony Cyril Scott
RWTH-Aachen University
Read the Original
This page is a summary of: Numerics of the generalized Lambert
W
function, ACM Communications in Computer Algebra, July 2014, ACM (Association for Computing Machinery),
DOI: 10.1145/2644288.2644298.
You can read the full text:
Resources
Contributors
The following have contributed to this page