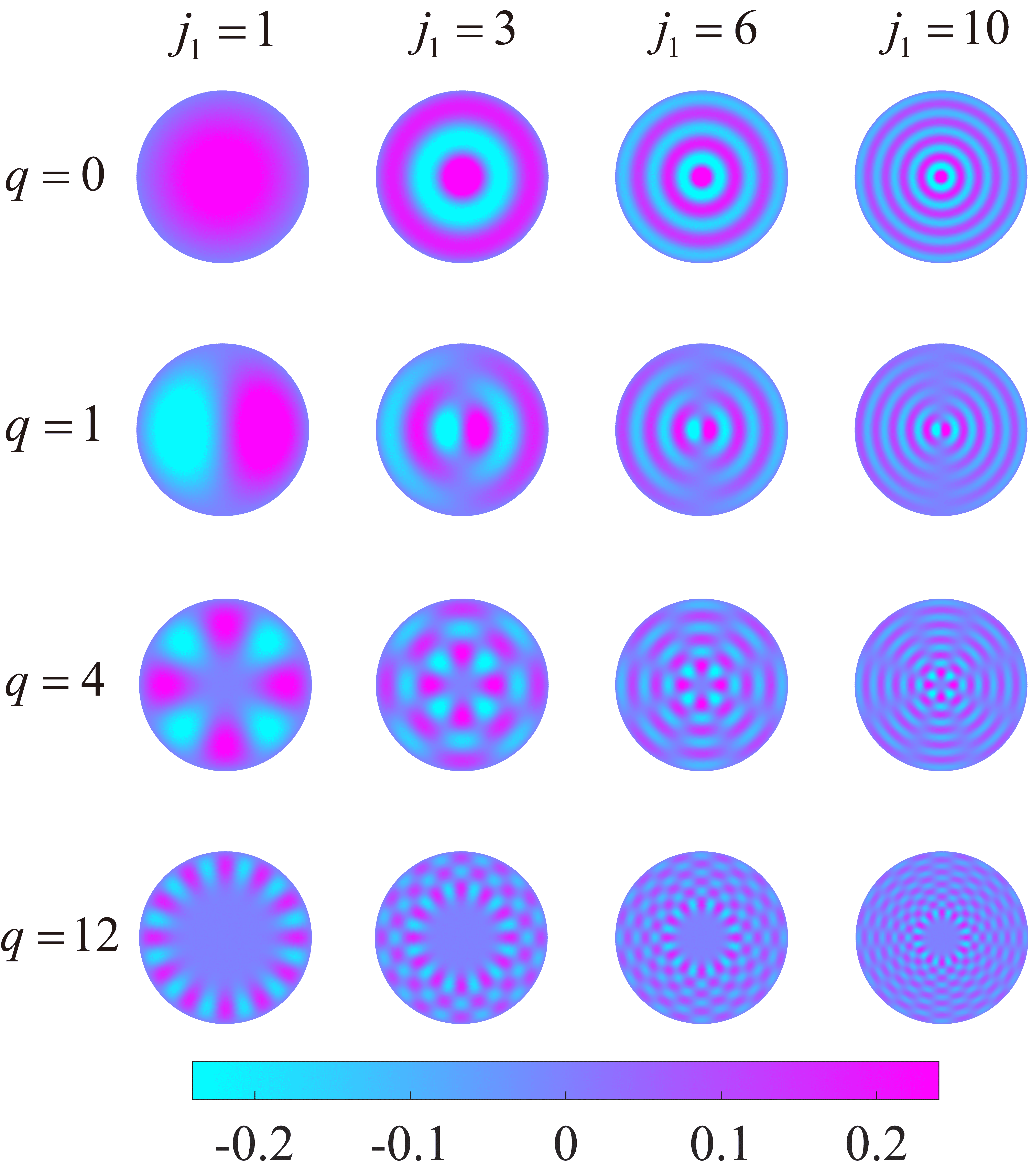What is it about?
We develop a new Monte Carlo method to simulate nematic liquid crystals. Our method can model thermal fluctuations that match with the equipartition theorem and also agree with molecular dynamics simulations. This method can be used to model thermal motion of topological defects and inclusions.
Featured Image

Photo by Abstral Official on Unsplash
Why is it important?
Conventional Monte Carlo method perturbs the director field of a liquid crystal locally, and therefore under samples long-wavelength modes. We overcome this shortcoming by sampling the director field in the Fourier space. Our method can be further used to accurately model thermal motions of topological defects and inclusion particles.
Perspectives

There are two theoretical difficulties in liquid crystal theory and simulation. One is to model thermal motions of topological defects and inclusions. The reliable method is molecular models, which suffer limited length and time scales. The other difficulty is to find the ground state of a liquid crystal structure. Available methods tend to be trapped in the local minimum states. Our Fourier-space Monte Carlo method shows promise in addressing these issues by sampling or perturbing the liquid crystal director field in its Fourier space. In other words, long-wavelength fluctuation modes can be well sampled. We show that this new method can match with the equipartition theorem and also molecular dynamics. Moreover, it can provide a new strategy to find global free-energy minimum states.
Rui Zhang
Hong Kong University of Science and Technology
Read the Original
This page is a summary of: Fourier-space Monte Carlo simulations of two-dimensional nematic liquid crystals, The Journal of Chemical Physics, November 2024, American Institute of Physics,
DOI: 10.1063/5.0231223.
You can read the full text:
Contributors
The following have contributed to this page