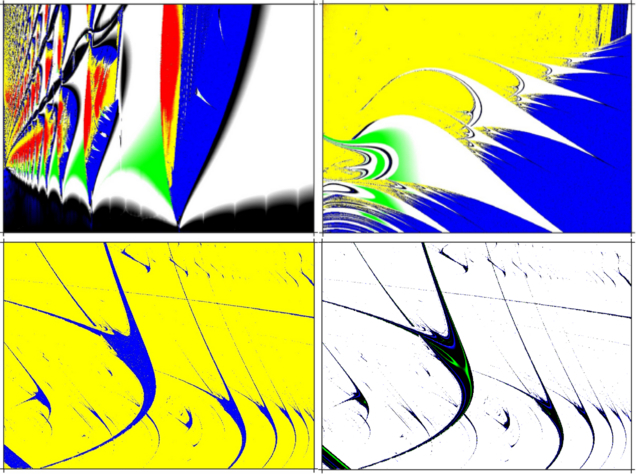
What is it about?
In the context of technological applications using mechanical devices, mathematical models and computational simulations are essential tools for characterizing dynamic behaviors and providing an overview of possible scenarios for desirable systems designed for practical purposes. Our work offers a dynamic characterization of a mechanical oscillator with intrinsic coupling from mathematical modeling and computational experiments. These types of systems have been used as the basis for various studies, ranging from synchronization phenomena to the development of energy-harvesting devices. As a key finding, in addition to periodic and chaotic oscillations, we identify a distinct nonlinear phenomenon composed of quasi-periodic behaviors associated with shrimp-shaped domains, which exhibit typical fractal properties such as self-similarity and highly organized patterns. Hitherto, shrimp-shaped domains have typically been observed in periodic solutions.
Featured Image

Photo by Solen Feyissa on Unsplash
Why is it important?
Our findings provide deeper insights into nonlinear dynamical behaviors in coupled systems, highlighting the importance of quasi-periodic solutions for oscillators with intrinsic coupling interactions. In nonlinear mechanics, intrinsically coupled oscillators represent a significant class of systems with technological applications. From a theoretical point of view, these oscillators have garnered substantial attention due to their complexity, which involves the coexistence of attractors - where multiple stable long-term behaviors exist for the same system under identical conditions, depending on the initial state of the system - fractal basin boundaries implying uncertainty in the final state, and chaotic oscillations compromising predictability.
Perspectives

Our contribution offers an alternative insight for both theoretical and experimental approaches, motivating researchers in nonlinear dynamics to investigate coupled oscillators from a new perspective. Quasi-periodicity has the potential to become a primary focus for studying complex dynamics, with implications for technological applications and a deeper understanding of phenomena such as synchronization.
Prof. Dr. Silvio L.T. de Souza
Federal University of Sao Joao del-Rei
Read the Original
This page is a summary of: Quasiperiodic shrimp-shaped domains in intrinsically coupled oscillators, Chaos An Interdisciplinary Journal of Nonlinear Science, December 2024, American Institute of Physics,
DOI: 10.1063/5.0234904.
You can read the full text:
Contributors
The following have contributed to this page